App-Rotary Tables Accuracy vs Radial Runout
| Accuracy vs Radial Runout |
|
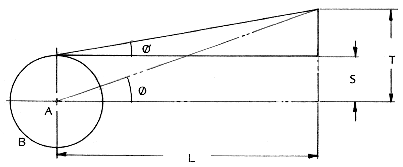
When rotary table turns in its bearings, it typically results in a radial run-out; essentially the roundness of the rotor rotation. For air bearings this can be as little as 1 micron T.I.R.; for some rolling contact bearings, it can be as high as 25 microns and more. This run-out is a linear translation along a plane which is perpendicular to the axis' centerline. Consider this geometry:
Where A is the theoretical center of the rotary table, and B is the run-out error. This run-out results in an offset error of ±S. If B is small relative to the part's tolerance band, it can be ignored. However, if B is large this error must be considered because the rotary table index position is generated about A; whereas, the part's index angle is generated from its own center, which is located on B. A part can not be centered better than the radial run-out value. If a table has a 10 microns run-out, the part can not be centered better than 10 microns. The smaller the radius L, the greater will be the resulting difference between Θ and Θ', and the greater the rotary table index error. The following formula allows to calculate the effective angular error, based on the run-out error of the rotary table bering, and the diameter of the part or feature:
where Θe is in arc seconds, B is the run-out and D is the diameter, with both being expressed in either inches or millimeters |